Brief Look into Measure Theory 🌱

A sigma algebra is a special collection of specific subsets of a set \(X\) that satisfy 3 following properties:

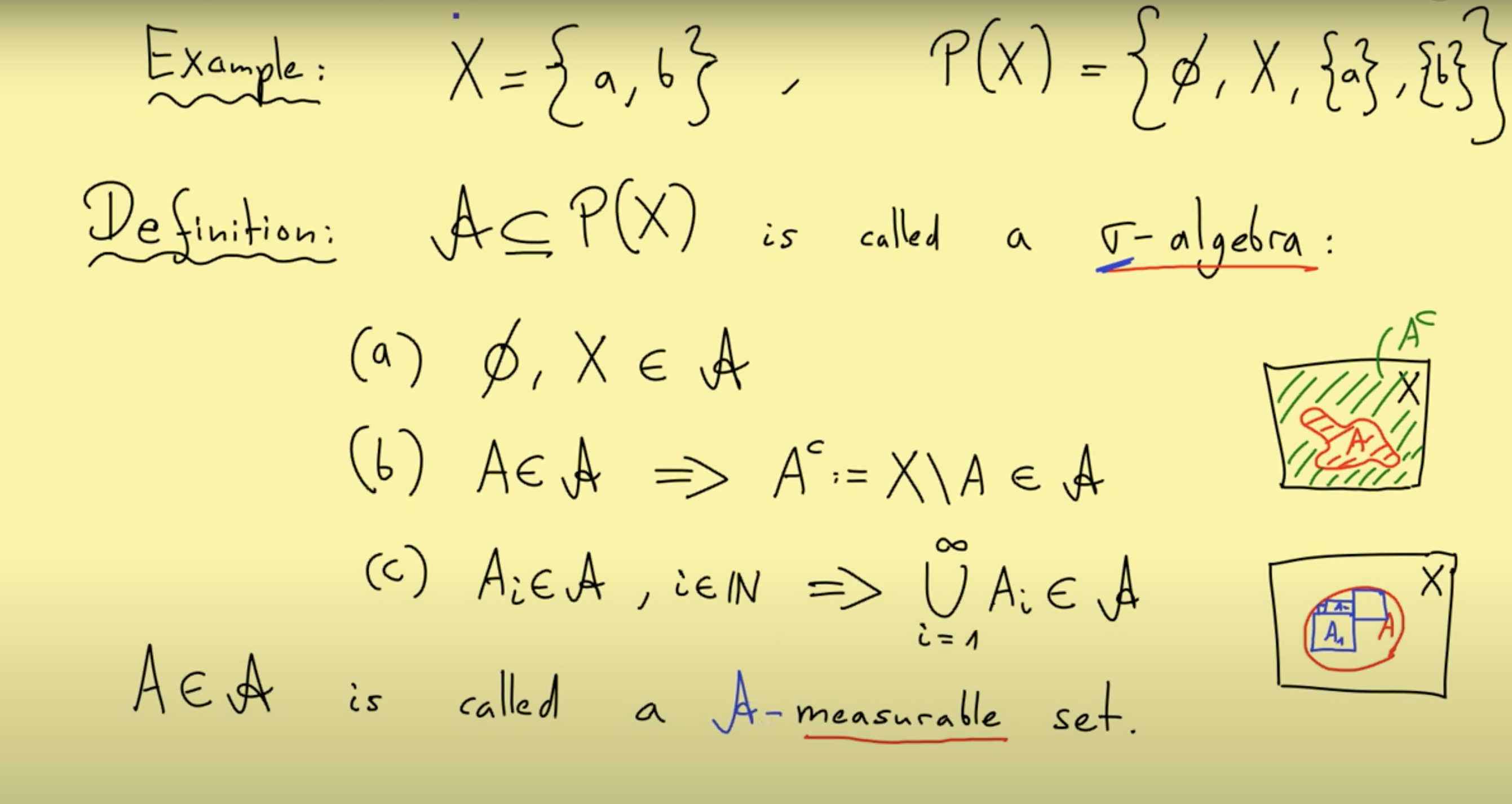
We can then define a measure map as the following:

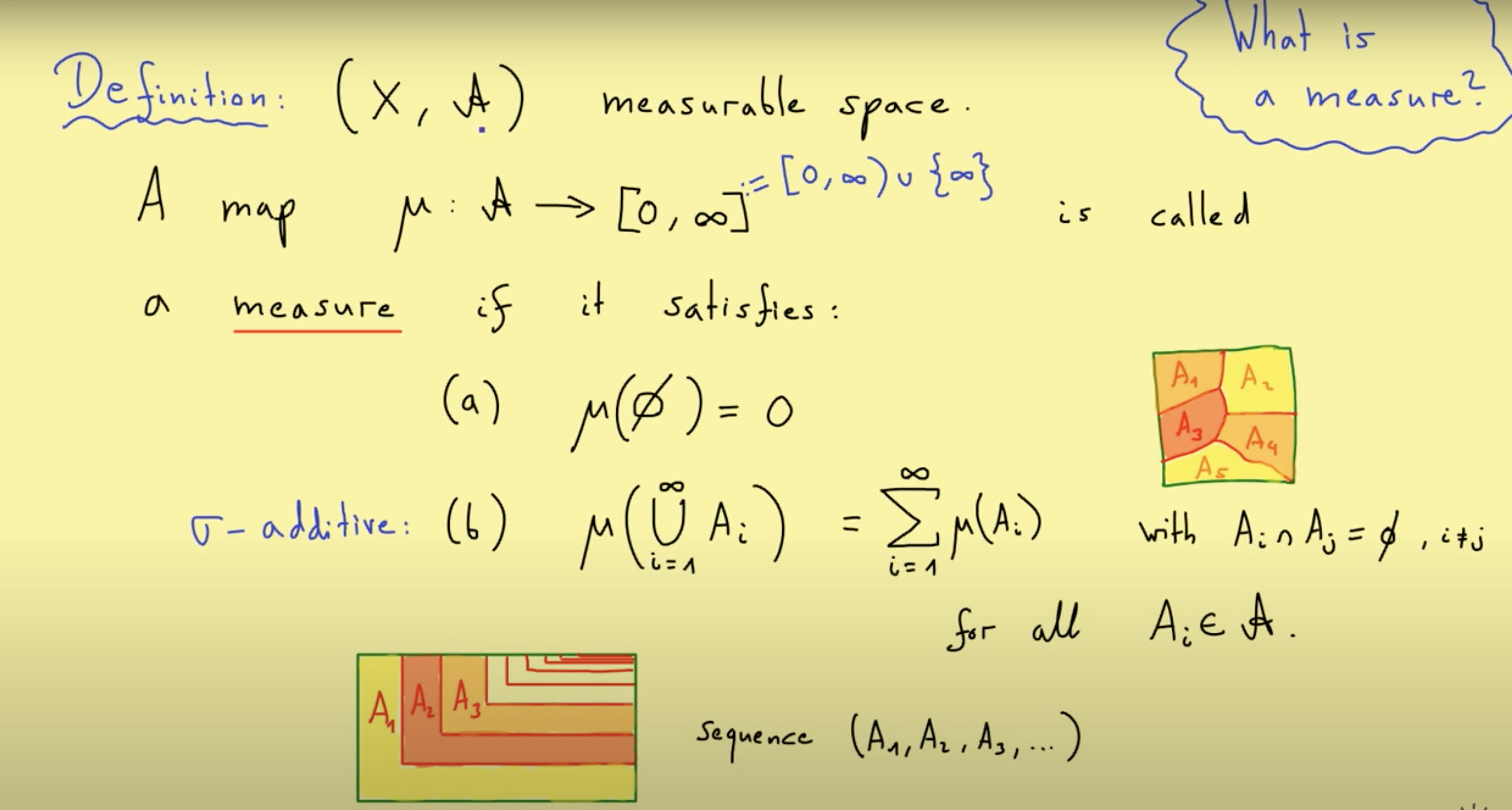
Here are some examples of measures:

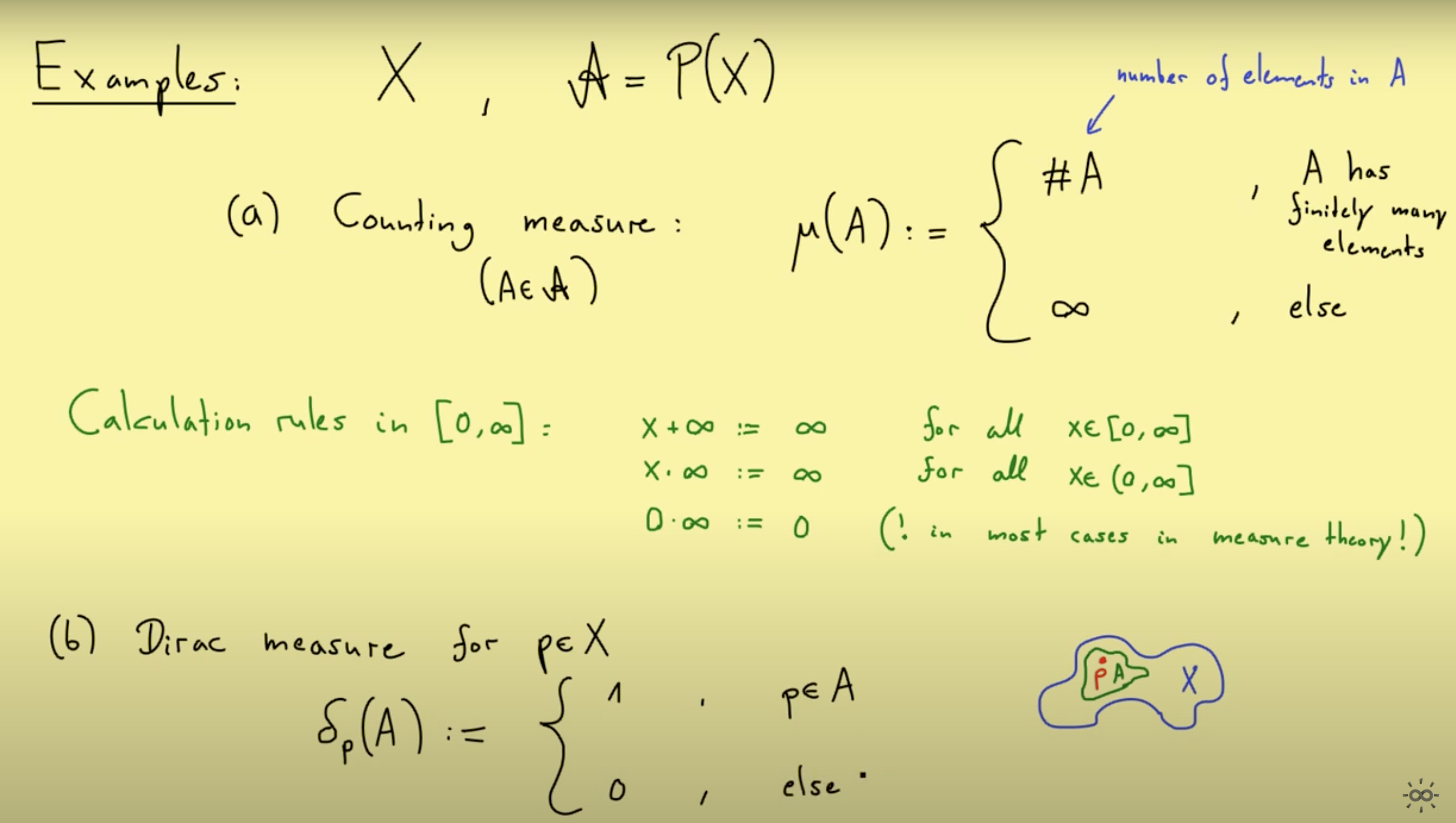
We can say a function mapping is measurable if the following condition holds:

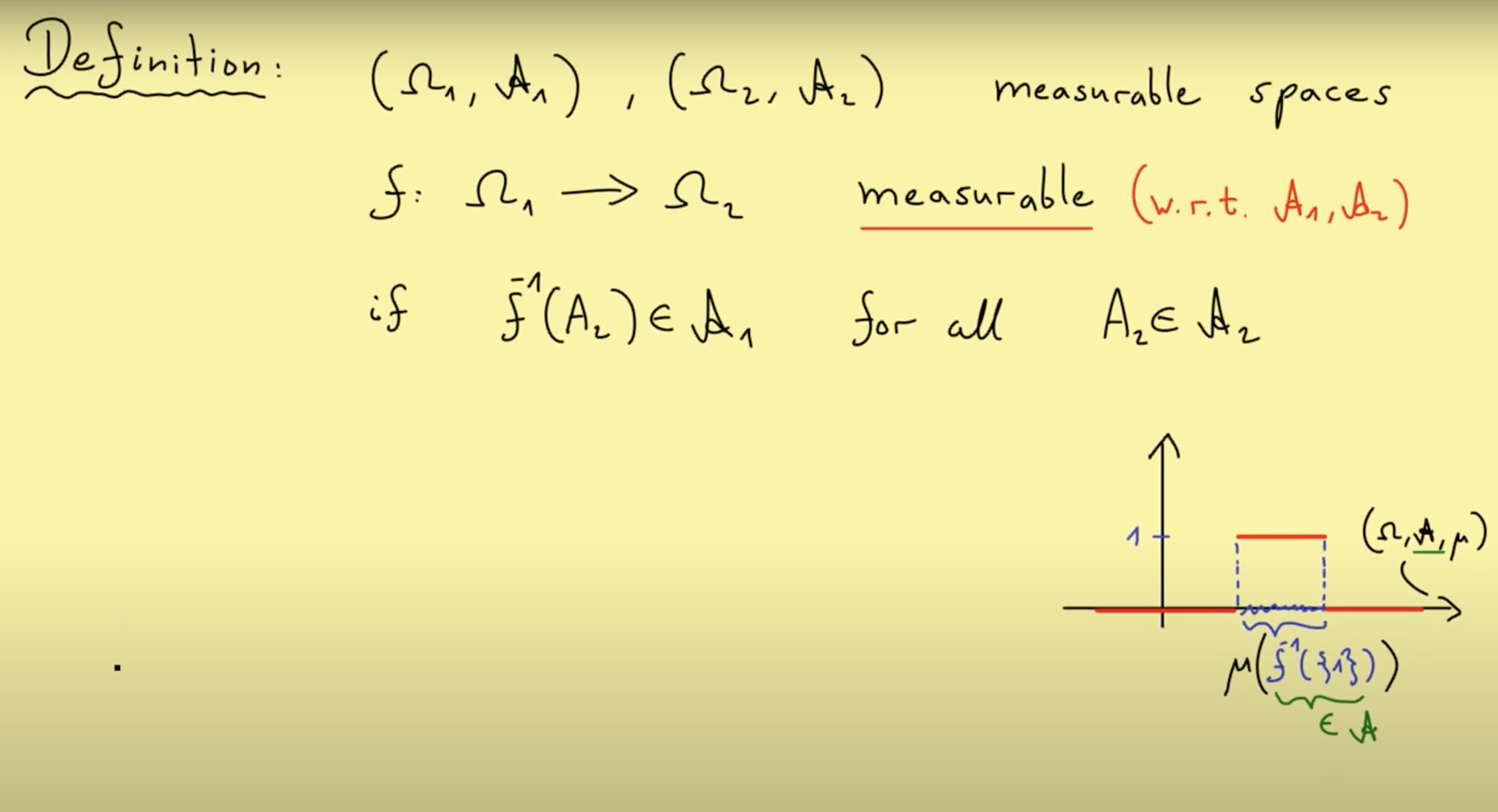
Lebesgue Integral

Problems with the Reimann Integral

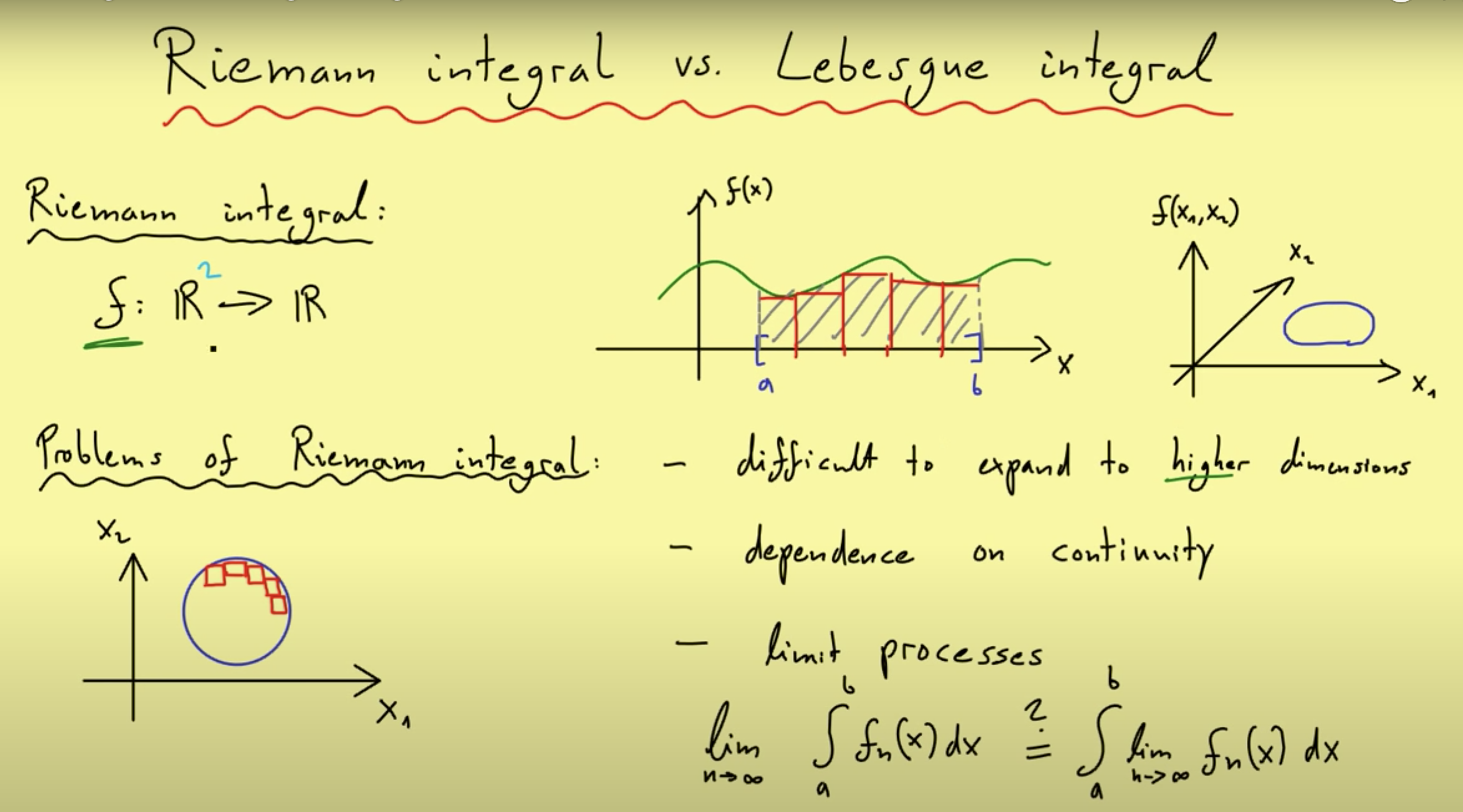
Reimann vs Lebesgue Integral

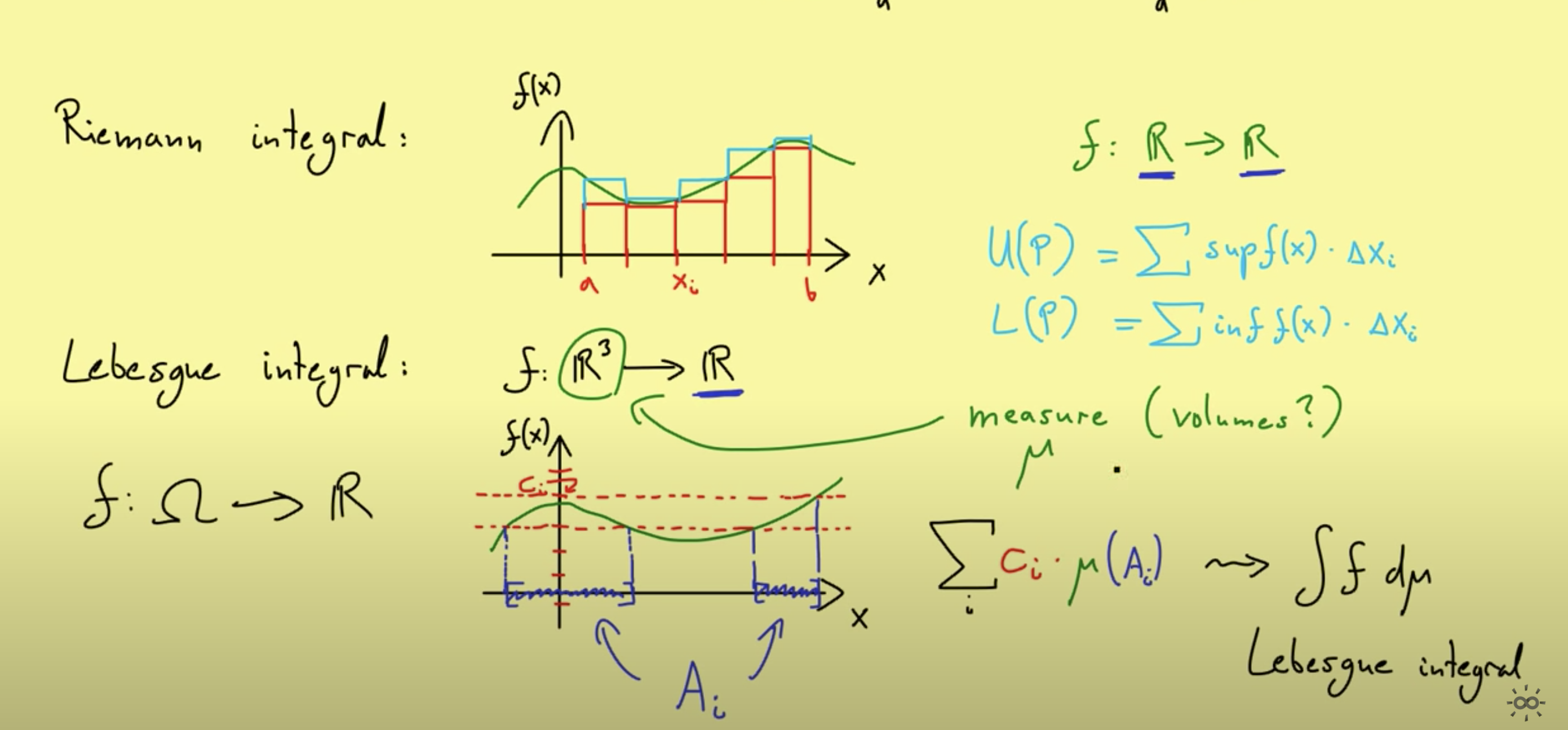
Defining the Lebesgue Integral

For simple (step, staircase, …) functions the Lebesgue Integral is defined as follows (where $\chi_A$ is some function mapping $x$ to the real numbers and $I(\chi_A) = \mu(A)$:

Generally, it is defined as follows:

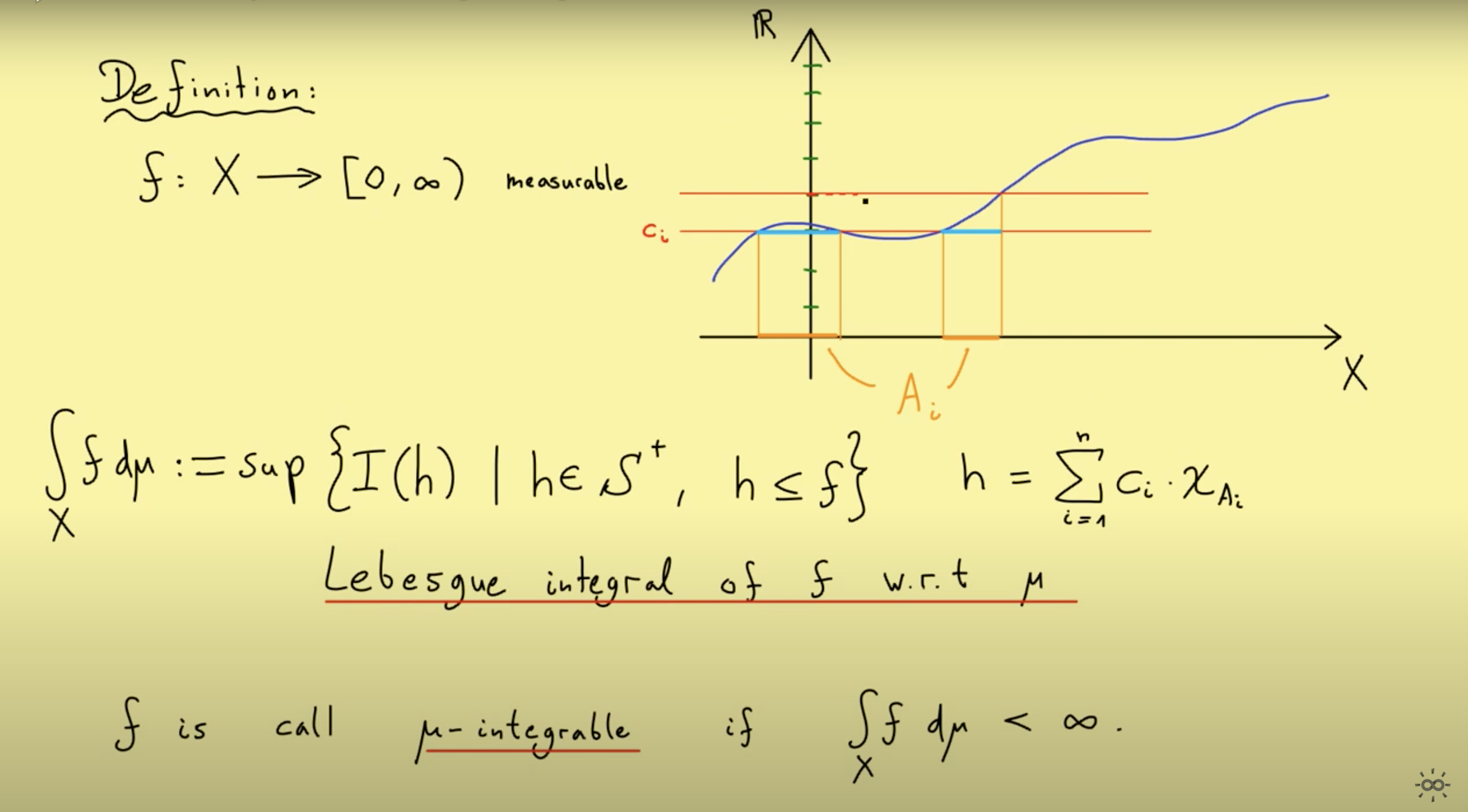
The integral of a measurable function $f$ is the supremum of the set of all integral values for step functions that lie below the function $f$.